The mathematical relationships which describe the phenomenon of doppler shiftt are relatively straightforward ones, for objects which are moving much slower than the speed of light. Examine the picture which follows which shows a wave traveling to the right with velocity v, wavelength lambda, and period T.

The period T, is the time it takes the wave to complete one cylce which must then be the time it takes for the wave to travel one wavelength. Since the velocity of the wave must be the distance per time, we can write the velocity of the wave as:

Since the period of a wave is the time it takes to complete one cycle, we can caluclate the number of cycles in a given unit of time, called the wave frequency, by taking the inverse of the period.

These two equations can be combined into what is called the universal wave equation:

The actual and perceived frequency of the siren are the same so long as both are at rest and are given by the formula above. However, if the siren is moving towards the stationary observer, then the distance between successive wavecrests is reduced by the distance traveled by the source during one period.

This resulting decreased wavelength called "lambda prime" l' is equal to:

where

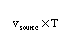
is equal to the distance traveled by the source in one period T.

The perceived frequency by the observer, which we will label f' must then be given by the following relationship:

The observer behind the truck, on the other hand, perceives a decrease in the frequency and increase in wavelength of the siren. (Remember, the frequency of the siren is unchanged.) This is due to the fact that the source moves away and the distance between sucessive wavecrests is increased due to the velocity of the source. As the siren is moves away from the stationary observer, the distance between successive wavecrests is increased by the distance traveled by the source during one period.

which results in a perceived lower frequency f' which is again given by

Without much additional thought, as I know you have been thinking for a while now, you could easily convince yourself that the doppler shift will occur under any of the following circumstances:

• The source is approaching a stationary observer.
• The observer is approaching a stationary source.
• The source and the observer are moving towards one another.
• The source is moving away from a stationary observer.
• The observer is moving away from a stationary source.
• The source and the observer are both moving away from each other.
• The source and the observer are moving in the same direction at different speeds.

You should also be able to easily convince yourself that the shift will yield an increase in the perceived frequency whenever the source and the observer are approaching one another, and a decrease in the perceived frequency whenever the source and the observer are moving away from each other.

So....here's the big question. Does light doppler shift? You probably remember the spectrum of visible light as ROYGBIV. So if the doppler shift works also for light then it must be possible to move so quickly towards a red traffic light that it would appear green to you! You might find it clever to use this argument if you get stopped for running a red light. However, the police officer might in turn charge you not for running the red but rather speeding. You might find it fun on your own to calculate your fine for travleing the speed necessary to make the light appear green to you, if the fine is $1 per mile per hour over the limit and you are traveling in a 25 mph zone. (You can do this problem in the Doppler Quiz which is linked to this site.)

Well, we guess you have discovered by now that light (or any part of the electromagnetic spectrum) can be shifted up in frequency or down depending upon your relative motion. In fact, if your recession velocity is great enough away from a visible light source, you could in theory warm yourself as you would be able to shift to the infrared or heat area of the electromagnetic spectrum.

Now take a moment to consider the diagram that follows:

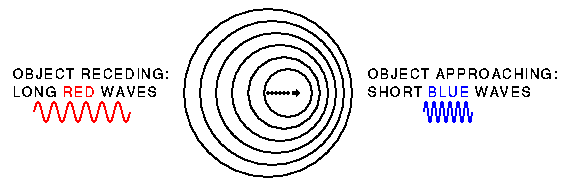
You will recognize it as the same as the fire truck approaching the stationary observer except now the source is light instead of sound. Notice that the right region where a perceived increase in the frequency is noticed is referred to as "blueshifted", and the region which would appear to be of a lower frequency to an observer on the left is referred to as "redshifted." And, it is important to note that the equations which were derived for sound will work equally well for moving light sources provided the light sources are not moving near the speed of light. (We would have to take relativistic effects into account at speeds approaching the speed of light.)

	Click here to see examples of redshifted spectra for some galaxies.
	Click here to return to more information on the Doppler shift (and a quiz!).